what is fourier transform

Fourier transform of discrete signals is continuous over the frequency range 0 f s 2. If a sine wave decays in amplitude there is a smear around the single frequency.

Fourier Transform Table

So yes ASP uses Fourier transforms as long as the signals satisfy this criterion.

. The ability to detect and measure almost any gas combined with the robustness and reliability of the technology makes FTIR ideal for a wide variety of applications such as emissions monitoring. Xxxiv and and are sometimes also used to. Fω 1 2π Z dtfteiωt 11 3 Example As an example let us compute the Fourier transform of the position of an underdamped oscil-lator.

The Fourier transform is a generalization of the complex Fourier series in the limit as. Thus from a computational standpoint this transform is not suitable to use. However it should be remembered that DFT and Fourier series pairs are.

The convergence criteria of the Fourier transform namely that the function be absolutely integrable on the real line are quite severe due to the lack of the. Fourier analysis converts a signal from its original domain often time or space to a representation in the frequency domain. Then change the sum to an integral and the equations become.

In practice discrete Fourier transform DFT is used in place of Fourier transform. The notation is introduced in Trott 2004 p. Imagine playing a chord on a piano.

DFT is the equivalent of Fourier series in analog domain. For others it is like holding a prism in a beam of sunlight and seeing what it contains a rainbow of colors. The word decomposing is crucial here.

The term Fourier transform refers to both the frequency domain representation. They are widely used in signal analysis and are well-equipped to solve certain partial differential equations. The discrete Fourier transform is an invertible linear transformation.

Today Fourier transforms are prevalent in many areas of science and engineering. The theory of Fourier transforms is applicable irrespective of whether the signal is continuous or discrete as long as it is nice and absolutely integrable. Fourier Transform - Properties.

With denoting the set of complex numbers. In other words for any an N -dimensional complex vector has a DFT and an IDFT which are in turn -dimensional complex vectors. When played the sounds of the notes of the chord mix together and form a sound wave.

The Fourier transform is an integral transform widely used in physics and engineering. Replace the discrete with the continuous while letting. Is called the inverse Fourier transform.

An animated introduction to the Fourier TransformHelp fund future projects. FTIR stands for Fourier Transform Infrared spectroscopy. The FourierTransformcan either be considered as expansion in.

A Fourier Transform of a sine wave produces a single amplitude value with corresponding phase not pictured at a single frequency. The Fourier Transform is a tool that breaks a waveform a function or signal into an alternate representation characterized by the sine and cosine functions of varying frequencies. For some it is the magic of seeing everything as waves.

G t g t gt is a new function which doesnt have time as an input but instead takes in a frequency what Ive been calling the winding frequency In terms of notation by the way the common convention is to call this new function. The Fourier transform of a function of x gives a function of k where k is the wavenumber. They are used in processing many of the signals we encounter in our everyday lives such as phone and TV signals and even in the evolution of the Stock Market.

Fourier Transform theory is essential to many areas of physics including acoustics and signal processing optics and image processing solid state physics scattering theory and the more generally in the solution of differential equations in applications as diverse as weather model-ing to quantum eld calculations. A discrete Fourier analysis of a sum of cosine waves at 10 20 30 40 and 50 Hz. The Fourier transform reveals a signals elemental periodicity by decomposing the signal into its constituent sinusoidal frequencies and identifying the magnitudes and phases of these constituent frequencies.

Its inverse is known as Inverse Discrete Fourier Transform IDFT. The Fourier transform is called the frequency domain representation of the original signal. The Fourier transform of an intensity vs.

The Fourier transform is a mathematical function that can be used to find the base frequencies that a wave is made of. It is a powerful gas measurement technology for simultaneous measurements of multiple gases. To overcome this shortcoming Fourier developed a mathematical model to transform signals between time or spatial domain to frequency domain vice versa which is called Fourier transform.

This works because each of the different notes waves interfere with each other by adding together or. It is a way of taking a signal or a function and deconstructing it into a series of sines and cosines. A fast Fourier transform FFT is an algorithm that computes the discrete Fourier transform DFT of a sequence or its inverse IDFT.

Fourier transform is a mathematical transform that decomposes functions depending on space or time into functions depending on spatial or temporal frequency such as the expression of a musical chord in terms of the volumes and frequencies of its constituent notes. The Fourier transform FT decomposes a function of time a signal into the frequencies that make it up in a way similar to how a musical chord can be expressed as the frequencies or pitches of its constituent notes. Fourier transform has many applications in physics and engineering such as analysis of LTI systems RADAR astronomy signal processing etc.

What is the Fourier transform really. For some others it is a tool to visualize what a piece of sound recording contains and adjusting it to make it sound better. The Fourier transform teaches us to think about a time-domain signal as a waveform that is.

However it is perhaps more common to talk about Laplace transforms which is a generalized Fourier. The Fourier transform of a function of t gives a function of ω where ω is the angular frequency.

Fourier Transform Google Search Physics And Mathematics Learning Math Math Methods

Fourier Transform Google Search Physics And Mathematics Learning Math Statistics Math

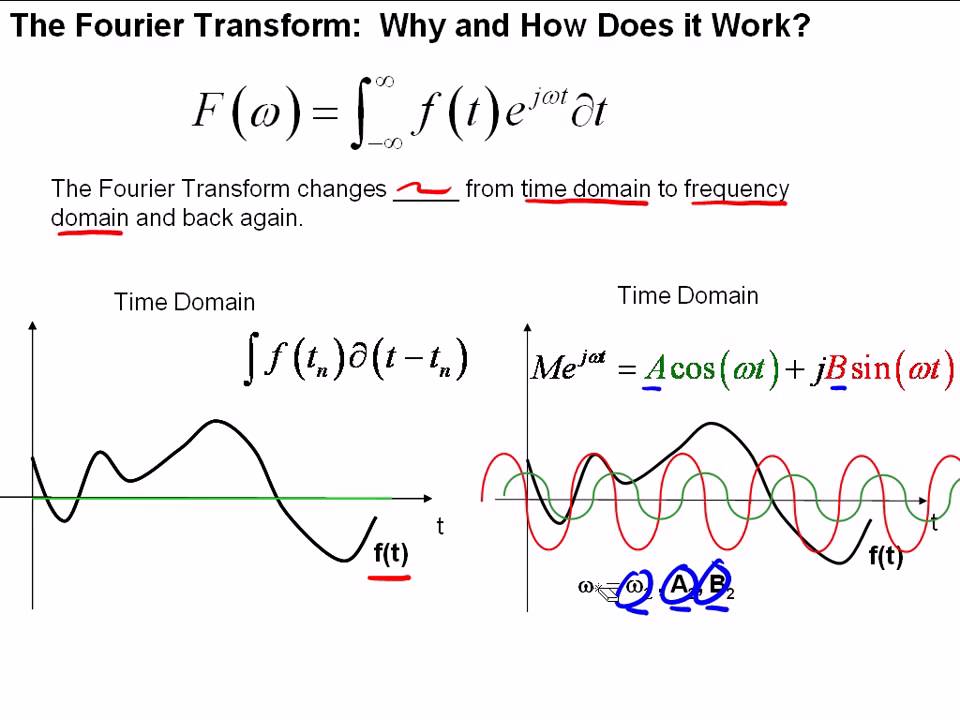
Electrical Engineering Ch 19 Fourier Transform 1 Of 45 What Is A Fourier Transform

Fourier Transform Table

Understanding The Fourier Transform

Fourier Transform Wikipedia The Free Encyclopedia Transformations The Unit House Wiring

In This Third Part Our Goal Is To Understand How A Sinusoidal Signal Is Transformed From The Time Discrete Fourier Transform Signal Processing Transformations